Why present day IOL box A constant based on thin optics concept needs to be reworked to improve IOL power prediction with thick lens formulae
- Subhabrata Bhattacharya

- Apr 23, 2024
- 7 min read
Updated: Apr 24, 2024
Objective of this article: In this article I am proposing a method that helps manufacturers as well as users adapt IOL box A constant to that of A constant applicable to thick lens paraxial IOL calculation formulae.
Background:
The application of constants in IOL power calculation is not a new concept and has been present ever since Fyodorov introduced his theoretical thin optics based IOL calculation formula in 1967. The constant that Fyodorov and other theoretical formulae used was the anterior chamber depth (ACD), that represented a value that helped predict the IOL position, post operatively. Though theoretical formulae, back then, predicted the power of anterior chamber IOLs, it underscored the importance of use of constants in the formula that would help arrive at the IOL power based on predicted post operative IOL position. It is worthwhile to note that these first generation of theoretical thin lens formulae, used a fixed value as constant for a specific IOL of a manufacturer.
The idea, that IOL constants may have to be adjusted according to patient’s axial length (SRK II), and later with Keratometry of the patient (SRK T), was a relatively new idea conceived in late 80s and early 90s. This was popularized by the need to predict IOL position accurately with the advent of posterior chamber IOL positioned in the capsular bag that would contract postoperatively. While the SRK II formula adjusted the manufacturer constants with the axial length of the patient, the SRK T, a combination of regression and theoretical thin lens paraxial formula further adjusted the manufacturer’s constants with corneal reading.
A constant with thin and thick lens formulae-
The concept of constants was introduced when thin lens theoretical formulae were the only ones available for IOL calculation. In Gaussian optics, a thin lens is such that its thickness is so negligible that all refraction is considered to happen only at one plane. The thin lens concept does not consider optical aberrations or dispersion (chromatic aberration) of light. The thin lens has two refracting surfaces; however, the lens thickness is so negligible that all its refraction is considered to happen at one plane. Or in other words, the thickness of the lens is disproportionately smaller than its radius of curvature. Biometry formulae like Fyodorov (1975), Colenbrander (1973), Binkhorst (1975) were thin lens theoretical vergence formula. The SRK T is a modified thin lens formula based on its regression based parent formula, the SRK. Thus modern day IOL calculation formulae like the SRK T, Hoffer Q and Hagis, consider the cornea and lens as thin lens with single refracting surface. The Gaussian thin lens and thick lens formula has been described in detail at quickguide.org (Gaussian optics, Gullstrand eye, & Biometry- https://www.quickguide.org/post/all-about-gaussian-optics-gullstrand-eye-theoretical-and-ray-tracing-formula )
In contrast, a thick lens formula considers the lens optic to have separate pairs of refracting planes - one anterior and the other posterior refracting plane. The object and image side focal length is calculated with respect to these refracting planes.
With the advent of thick lens paraxial formulae like Barrett Universal, the need to revisit the manufacturers box A constant is important, as simple assumptions of theoretical thin lens formula is no longer applicable. For example, the A constant in the SRK T formula is the distance between the corneal vertex and the anterior vertex of the IOL. This is often referred to as ALP or actual lens position.
In predicting the postoperative lens position or ALP, SRK T adopts corneal height formula, which is a combination of corneal curvature, corneal width (White to White) and an offset factor to account for lens position from iris plane after implantation (this is explained in the article ‘Understanding Biometry & IOL Calculation Formula’ - https://www.quickguide.org/post/biometry-iol-formula. When the formula was developed in the early 1990s, there was very little means of knowing corneal width in an average practice. Therefore, regression-based formula was applied to understand corneal width and then apply a theoretical formula to derive corneal height. This part is explained in my article mentioned above.
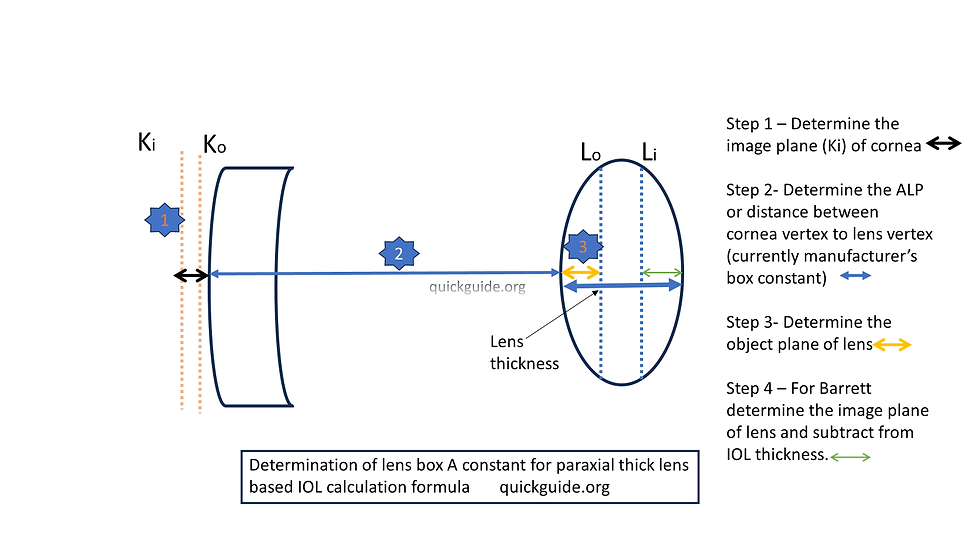
The lens position in the theoretical thick lens-based formula is the distance between the image plane of cornea (not anterior vertex of cornea) to the first principal plane of the IOL (fig 1). Thus applying the thin lens A constant concept calculated by manufacturers based on distance between vertex of cornea to anterior IOL position, and directly adapting it to thick lens formula leave scope for error. The cornea is a meniscus shaped lens, thus the principal planes of the cornea, at least the image plane is not inside, but anterior to the cornea. Several authors have noted that the shape of the average cornea being considered, the image plane of the cornea should be .05 mm anterior to cornea. With steeper and flatter cornea, this value may change, but not grossly.
The first principal plane of the IOL, that is the object plane of the IOL, is the final IOL position that may be referred to in a theoretical thick lens formula (ELPt). However, matters are complicated, as some thick lens formula, like Barrett, use the second principal plane as reference plane to the final IOL position. Barrett is an unpublished formula. In my understanding, Barrett uses second principal plane as reference point, as this plane in a lens remains largely the same with change in IOL geometry as you move from low power to higher power IOL of the same manufacturer. Thus, lens box A constant for Barrett may have to be defined as the distance between the image plane of the cornea to the second principal plane of the lens.
The image plane of the cornea, and the object and image plane of the lens is not difficult to find out. If we know the anterior and posterior radius of curvature of the cornea, the refractive index of cornea (1.376), the thickness of the cornea, and apply the refractive index of air and aqueous, we will know the image plane of the cornea. For example, the image plane of the cornea will be -.057 mm before the cornea, with anterior and posterior radius of corneal curvature being 7.8 and 6.8 mm, thickness of cornea 555 micron, and with refractive index of cornea and aqueous being taken as 1.376 and 1.336, respectively (Fig 3).
Similarly, knowing the anterior and posterior radius of curvature of IOL, the refractive indices of IOL, aqueous and vitreous, and the thickness of IOL, will help to get the first and second principal planes of the cornea. My own calculator available at quickguide.org helps you to find this (https://www.quickguide.org/post/thick-lens-formula) . With an anterior and posterior radius of curvature of 16.379 and -25.0 mm, refractive index of 1.55 and a central optic thickness of .7 mm the first and second principal plane are at .239 and -.365, respectively.
The lens position in the theoretical thick lens-based formula is the distance between the image plane of cornea (not anterior vertex of cornea) to the first principal plane of the IOL (fig 1)
Knowing such values of the principal plane will help us to arrive at the A constant of an IOL for the thick lens, both for conventional thick lens formula as well as for Barrett. For Barrett, currently the manufacturer box A constant is converted to Lens Factor, a distance from the iris plane to the second principal plane of the IOL. If box A constant is re worked to represent the effective lens position of a thick lens, IOL power prediction may improve. It is important to point out that Graham Barrett did study the principal plane of a particular widely accepted hydrophobic IOL of an esteemed manufacturer. But not all lenses of this manufacturer was studied, neither is there a scope to study all lenses of different manufacturers across the continents. Hence the importance of the approach being described in this article.
Once the first principal plane of the IOL is determined, adding this value with the distance between the image plane of the cornea and anterior lens position will give us the effective lens position of the IOL adapted for the thick lens formula. For Barrett however, an extra step is involved (Fig 2) . Since Barrett considers ELP of the thick lens as distance till the second principal plane of IOL (image plane of IOL), adding the distance between the first principal plane from the vertex of the lens and the distance between the first and second principal plane will help us to arrive at the total distance of second principal plane from the lens vertex. Alternatively, one may substract the second principal plane from the total thickness of the IOL to arrive at this distance. Thus if the lens thickness is .7 mm and the second principal plane is at a distance of -.365 mm, substracting the two would give us a value of .335. Thus the second principal plane is at a distance of .335 mm from anterior lens vertex.
Thus the A constant for the thick lens that considers effective lens position till the second principal plane (Barrett) will be .057 + Aconstant according to think lens box constant + .335. If the thin lens box A constant is 119.0, the new A constant for thick lens (ELPt) will be 119.39 for the Barrett formulae.
However, for thick lens formula that considers effective lens position till the first principal plane, the new A constant will be .057 + box A constant +.239, that is for the box A constant as 119.0, the new constant will be 119.296 ( rounded to 119.3 )
What could be a drawback with this approach-
While the above method may help improve refractive outcome, by marrying current box A constant to that of a thick lens formula, yet we have to keep in mind that most thick lens formulae are not truly thick lens formulae. Many of them are hybrids or either conseder the lens or cornea as thick lens (and not both), may use fudge factors to marry thick lens formulae to current generation manufacturer box A constant.
Thus, ideally manufacturers should give a list containing all IOL parameters to IOL formulae authors (preferably diopter wise) to help them study the lens geomentry, and then adapt constants that will work with the lenses for refractive outcome. Practically, this is extremely difficult on both sides. Currently, formulae like the K6 (David Cooke and Timothy Cooke) or the Barrett Universal to the best of my knowledge have developed such formulae that are based on studing of one lens optical property only. Manufacturers may approach such authors to help them study their IOL geometry across the dioptric range and help adapt the thick lens formula to particular IOL models.
Conclusion:
With this article, I hope I could explain how manufacturer provided box A constant can be adapted to thick lens formula, to help determine the effective lens position of thick lens (ELPt). Prediction of the refractive target warrants determination of the lens position accurately. I believe that adapting the box Aconstant based on thin lens optics to A constant that suits the thick lens formula may help us to achieve refractive prediction for more number of patients.
Next: Keep an eye on an calculator in this website that will help you to adapt current box A constant to A constants that suit thick lens IOL power calculation.







